Dear Organlearners,
Dan Chay <chay@alaska.net> writes under the
Subject: Predator "experts" and expert predators LO21106
>But I couldn't pass up the opportunity to link "tyranny of experts"
>to competition.cooperation.predator.prey (fruitfulness).
Greetings Dan,
I love your intuition. Once you understand the dynamics of the
Digestor, you will be able to deal with the "tyranny of experts".
I am sorry, but I had to change your name for the subject for
reference purposes. It is a pity because your name for the subject has
an important truth to it: "the riddle we see in the mirror" (or "the
dog biting its own tail"). I do hope that the following account of the
Digestor will help you "to look through the mirror" (or "avoid chasing
what is not food").
I must stress that the Digestor is about evolutionary
self-organisation close to equilbrium where the entropy production is
low. Hence it complements revolutionary self-organisation far from
equilbrium where entropy production is high. Here, at the edge of
chaos, as a result of the high entropy production, ordinate
bifurcations will occur which may then result into constructive
emergences or destructive immergences. But what happens close to
equibrium? The Digestor is a model to tell us more about evolutionary
self-organisation.
In
Competition.Cooperation.Predator.Prey LO21007
< http://www.learning-org.com/99.03/0247.html >
I tried to motivate why we should look closer at equilibrium and not
fix our attention only on the edge of chaos. I also gave some
historical account of what led to the discovery of the Digestor. The
discovery itself began when I observed the determination of the
sulpate content of a sample by gravimetric analysis, an important
technique in analytical chemistry. Unlike on hundreds of previous
occasions, I saw a deeper message in the observation.
Now before some of you get the idea that we are now going to indulge
in theory with little practical value, let us see how important
sulphur (S) and its compounds are. Sulphuric acid (H2SO4) is probably
the most important compound used in the chemical industry at large.
When it dissolves in water, it forms H3O+ ions (which takes over as
the acid function through the leveling effect) and SO4-- ions as the
remainder. (One day I want to write on this leveling effect and its
importance to leadership.) Sulphur dioxide SO2 is the second largest
pollutant (next to carbon dioxide CO2). It is the main contributor to
acid rain with its devastating consequences. The element sulphur is
one of the four micro nutrients (S, Ca, Mg, Fe) to all life. There are
six elements (C, O, H, N, P, K) acting as major nutrients and various
other elements (B, Mn, Cu, Zn, Mo) acting as trace nutrients.
To determine the suphate content of a sample, the sample must be
brought into solution. This is often not an easy task. This solution
is then treated with another solution to form a precipitate (as
insoluble possible) which will contain the suphate ions. This
precipitate is then weighed so that the percentage of sulphate in the
original sample can be calculated.
The ion Ba++(aq) is used because barium suphate BaSO4 is very
insoluble, removing practically all suphate ions SO4--(aq) from the
solution (note the essentiality fruitfulness). The (aq) means that the
ion is in solution, i.e, surrounded by water (aqua) moleclues. The ++
say that the barium ion is a barium atom from which its two valency
electrons with one negative charge each have been removed. The -- say
that the cluster of one sulphur atom S and four oxygen atoms O has two
additional electrons.
Hence, when a solution containing barium ions Ba++(aq) is mixed with a
solution containing sulpate ions SO4---(aq), a thick, powdery
precipitate is immediately formed. This described by the formula
equation
Ba++(aq) + SO4--(aq) = BaSO4(s)
The entropy production is so high that equilibrium is reached after a
few seconds. Since the equilibrium is reached so quickly, the
precipitate is in a very finely divided state. There is no time for
crystals to grow. Thus, if we wish to obtain the precipitate by
filtration, most of it will run through the filter paper and not
remain on it.
However, the equilibrium state is meta-stable. The reason is as
follows: if we leave the mixture to stand for some weeks, the
precipitate changes from a powdery form to a crystalline form. Please
note that this happens very close to equilibrium. Should we filter it
again, all the precipitate will remain. This is because the crystals
have grown considerably in size while decreasing in numbers. The time
this process takes may be reduced to a few hours by increasing the
temperature of the mixture to just below the boiling point of water.
This process is known in analytical chemistry as Ostwald's digestion.
It has been researched quite extensively in terms of solubility,
crystal growth, etc., but not in terms of its basic thermodynamics.
How did the crystals grow in size? Did the smaller crystals merely
coagulated to form fewer and bigger crystal clusters? No. The smallest
crystals dissolved while the dissolved ions moved to the bigger
crystals to be repacked on their surfaces. In other words, the
smallest crystals acted as prey and the biggest crystals as predators!
To understand this process we must think in terms of a dynamical
equilibrium and not a static equilbrium.
What is a dynamical equilbrium? To understand it, we have to think
atomistically and not macroscopically. Macroscopically all matter has
temperature which is the result of thermal energy. On the level of
atoms we must distinguish between the different behaviours of solids,
liquids and gasses. In a solid the temperature corresponds to the
vibration of atoms. Otherwise the atoms are not free to move around.
In a solution it corresponds to molecules and ions crawling over and
bustling against each other. At the meta-stable equilbrium water
molecules H2O crawl of the surface of BaSO4 crystals. They pluck a few
of the Ba++ and SO4-- ions from the crystal surface into solution
while some other already solvated ions get trapped again at the
surface of the crystal.
The Ba++ and SO4-- ions are arranged in a regular pattern in the
crystal, called its lattice structure. The electrical charge of each
ion results in a electrical force field. Because the ions are arranged
in a regular pattern, these electrical forces add up in some
directions and cancel out in other directions. The resulting long
range forces may be called Madelung forces. The bigger the crystal,
the stronger the Madelung forces and hence the less soluble the
crystal. In other words, when a big and a small crystal compete for
the few Ba++ and SO4-- ions in the solution, the bigger crystals with
their stronger Madelung forces will win.
Do you already, as Jon Krispen often describes it, see the lights
coming on and hear the bells ringing in your mind? Wait, the truely
remarkable part has still to come.
The mere size of a crystal is not the only determining factor.
Pefectly formed crystals, including diamonds, are very rare in nature.
When any kind of crystal is formed, they contain defects (faults,
errors). A crystal defect is nothing else than a break in the regular
pattern of the crystal lattice -- a deviation from the perfect order.
The effect of any crystal defect is to reduce or impair the Madelung
forces at the surface of the crystal. There are five basic types of
crystal defects. Any number of each of these defects may occur in a
crystal. Thus a smaller crystal C1 with less crystal defects (errors)
in it will outgrow and eventually devour a bigger crystal C2 with more
crystal defects in it!
Let us symbolise this digestive process. Let m denote the quantitative
size of the crystal. The value of m is given by the number of ion
pairs in the crystal. Let M denote the qualitative order of the
crystal. The more perfect a crystal is (i.e, the less defects a
crystal has), the higher is its M value. Whereas m denotes the size of
the lowest order, M denotes the number of higher order levels. In
other words, m is a horisontal or extensive quantity while M is a
vertical or intensive quantity. Let us then consider a small crystal
C1 with values m1 and M1 as well as a larger crystal C2 with values m2
and M2. The fact that C1 is smaller (<) than C2 is symbolised by the
expression m1<m2. The fact that C1 is of a higher (greater, >) order
than C2, is symbolised by the expression M1>M2. Thus it is quite
possible that C1 will beat C2 despite m1<m2 because M1>M2. But, for
m1<m2, when M1<M2, there is no chance for the smaller crystal to make
it.
I have succeeded in deriving an equation for the free energy change of
the digestion reaction. It is given by
/_\F = - /_\n*m2*[E(m1, M1) - E(m2, M2)]
Here /_\F is the change in free energy when /_\n ion pairs move from
crystal C2 to crystal C1. Thus the factor /_\n is an entropic flux.
Furthermore, for example, the quantity E(m1, M1) is the energy E of
the Madelung growth of crystal C1 with m1 ion pairs and M1 crystal
perfections. The factor
E(m1, M1) - E(m2, M2)] clearly contains a difference and thus is
associated with the entropic force together with the factor mSU.
In order to bring this equation more into line with our systems
thinking, the crystal C1 will represent our system SY. All other
crystals C2, being systems themselves, will represent the surroundings
SU. In other words, we will now be studying the interaction close to
equilibrium between any system SU and its environment SU. The Digestor
is the whole setup and not merely the system SY. Thus the equation may
be rewritten as
/_\F = - /_\n*mSU*[E(mSY, Msy) - E(mSU, Msu)]
Unfortunately, it is not possible with ASCII text to present by
subscripts the indicators (qualifiers) "SY" and "sy" for system as
well as "SU" and "su" for surroundings. Hence, note that "SY", "sy",
"SU" and "su" do not represent quantities like F, n, m, M and E do.
Also note that the sign /_\ in /_\F symbolise the "difference" or
"change" between the initial and final value of the free energy F.
Remember that a system's free energy F is that part of its total
energy E which it can use to change its future organisation. By using
this free energy F, it produces entropy S. The entropy may be
dispersed as chaos (of becoming) and also be concentrated as order (of
structure). For any spontaneous change in organisation the system's
free energy must be used up. Thus spontaneous changes corresponds to a
negative change (decrease) in free energy, ie /_\F<0. When
non-spontaneous changes happen, the system's free energy F increases
so that the change in free energy is positive, i.e /_\F>0. Such
non-spontaneous changes have to be forced on the system by the
surroundings SU through working on the system SY.
This equation is actually simple. It contains only three factors,
namely /_\n, mSU and the E difference. (Newton's second law of
dynamics also contain only three factors!) Now what does this equation
tells us?
Firstly, the factor /_\n says that the more the elementary building
blocks (ion pairs) flowing between the system SY and the environment
SU, the more the change in free energy is also enhanced. The phrase
"elementary building blocks" means that we should not think omly in
terms of pairs of ions like Ba++ and SO4--. Many kinds of crystals are
made up of three or more different ions -- a fascinating study itself
in mineralogy. When we move from the Digestor model for crystals to
other kinds of evolutionary self-organisation, it is better to think
in terms of "elementary building blocks". For example, in education
the learning objectives (not goals) on a topic function as "elementary
building blocks".
Secondly, it is most important to note that the quantity mSU (the
quantitative size m in the lowest orders of the surroundings SU)
occurs twice in the equation whereas the related quantity mSY of the
system occurs only once. The second occurance of the quantity mSU is
as a factor. This makes the equation asymmetrical in mSU. The richer
the environment SU is in lower orders, i.e the greater mSU, the more
the change in free energy F is enhanced. It means that the "playing
fields are not level" in evolutionary self-organisation. The lower
orders of the system itself, expressed by mSU, do not play such a role
here. In other words, an environment rich in lower orders enhances the
system's free energy for digestion and thus evolutionary growth. An
environment poor in lower orders inhibits the change in free energy.
I have not yet made it plausable that the Crystal Digestor may be
applied to even human situations in which the abstract world of mind
plays an indissoluble role. But a few examples here of this
asymmetrical contribution of mSU (offsetting the base line) will help
us to understand what it means. As first example we may consider the
migration of people from rural areas to city centres. The m of a rural
area is lower than the m of a city area. People experience how they
can accomplish more in city areas -- the enhancement of their free
energy. Consider as second example the caste system (class system)
which occurs in almost all human societies since times immemorial.
People in a higher class (greater mSU) despise people from a lower
class entering their class because of a possible lowering of the mSU
of their class. A last example. Think of a computer application of
which its front page is loaded with hundreds of choices to make. This
complexity will inhibit the user's free energy -- it is said to be
"user unfriendly". To hide this complexity in order to make the m
value of the tasks smaller, a task bar has been invented. The task bar
shows only the categories of choices. By clicking on a category,
additional categories or the very choices themselves will be shown.
Thirdly, let us study the factor [E(mSY, Msy) - E(mSU, Msu)]. It is
made up by the difference between two similar terms for energy as a
result of order, the one for the system SY and the other one for the
surroundings SU. When the order in the system SY is greater than the
order of the surroundings SU, we will have
[E(mSY, Msy) - E(mSU, Msu)] > 0
so that /_\F < 0. In other words, when the system SY is more ordered
than the surroundings SY, the system will behave spontaneously,
working for its own self-organisation. But when ther Surroundings is
more ordered than the system, the system's organisation can only be
changed when forced by the surroundings to do so. However, as soon as
the surroundings SU stop working on the system SY, that organisational
change will not happen any more. Thus we say that the change was
non-spontaneous.
How do we know by means of the quantities mSU and mSY as well as the
qualities Msu and Msy when the order of the system SY will be greater
than the order of the surroundings SU? First of all, we have to know
the exact matematical form of the formula for the quantity E(m??, M??)
where ?? stands for SY or SU. Then we have to do calculations on a
variety of possibilities for each of the quantities mSY, Msy, mSU and
Msu. Lastly, we have to form an overall picture of the result so
obtained. We can represent the overall picture in terms of graphs as
follows.
[Rick, it is here where we need for the dialogue something more than
merely communicatiing in terms of ASCII based characters. I hope that
you or perhaps Doc Holloway will make it possible to make the
following three graphs available on the web. Fellow learners can then
click with their web browser on the URL to view these graphs. If such
browsing is not possible for somebody, send me a note and I will send
these graphs as file attachments to an email message.]
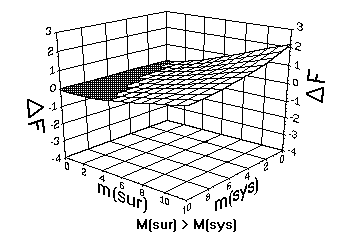
[Host's Note: Here are At's three graphs:
In order to repesent the influence of the four quantities mSY, mSU,
Msy and Msu on the fifth quantity, namely the change in free energy
/_\F. we actually need a 5th dimensional graph. This is not possible
in a three dimensional space. But because of the symmetries involved,
we may solve the problem somewhat by making use of three graphs. In
all three graphs the vertical axis represent the change in free energy
/_\F. If the change is negative (lowering of free energy, spontaneous
organising), the graph has a darker colour. If the change is positive
(raising of free energy, non-spontaneous organising), the graph has a
white colour. On the two horisontal axes the quantities mSY and mSU
are represented, namely the quantitative sizes of the system and the
surroundings in their lower level order.
But to represent the intensive quantities Msy and Msu concerning the
higher order qualities of the system and the surroundings, we have to
make use of three graphs. In the first graph with the smallest dark
coloured area, we have that Msy<Msu, i.e the system has less higher
order qualities than the surroundings. Note that the system is
spontaneous only in a small region close to the mSY axis where the mSU
values are small. To retain its spontaneous character, the system has
to grow into large mSY values. In the second graph with the
intermediate dark coloured area, we have that Msy=Msu, i.e the system
and the surroundings have similar higher order qualities. Note how the
system is spontaneous over a wider region. But the system still cannot
handel high mSU values and remain spontaneous. In the third graph with
the largest dark coloured area, we have that Msy>Msu, i.e the system
has more higher order qualities than the surroundings. Note that the
system is now spontaneous over a vast range of mSU values.
This digestive interaction of order between the system SY and the
surroundings SU in terms of mSY, mSU, Msy and Msu may be summarised as
follows. Observe the second graph for which Msy=Msu. Take the furthest
point of the graph from the origin into the fingers -- the corner
"coming out" of the 2D plane on which the graph is represented. To
simulate Msy>Msu (greater ordered system), pull the graph downwards so
that more of it falls below the base plane. The darker coloured area
will become larger. This latter case is the result when the system is
rich in higher ordered qualities, i.e after the system has experienced
a diversity of emergences. To simulate Msy<Msu (lesser ordered
system), pull the graph upwards so that more of it rises above the
base plane. The darker coloured area will become smaller. This latter
case is typical of a "disempowered system". The system cannot feed
upon the higher order qualities in the surroundings, but rather
becomes the prey itself.
These graphs representing the CHANGE in free energy as a result of the
interaction of order between the system SY and the surroundings SU,
can serve as the phsyical basis for the so called "fitness landscapes"
of the Santa Fe school of complexity thinkers (S Kauffman and J
Holland). For a fitness landscape we have to work with ABSOLUTE values
in free energy rather than relative changes. This means that the
graphs have to be inverted. Negative changes correspond to high free
energies and positive changes correspond to low free energies. Thus
the inversion has to raise the darker coloured areas and to lower the
white areas. By introducing different kinds of low level orders m and
different kinds of high level orders, a landscape similar to an
ordinary topograpic map, may be produced.
Obviously, up to now the Digestor is only valid for the competition
between crystals in the inanimate world. Thus we may have to speak of
it as the Crystal Digestor. Rather than looking for digestors in all
hierarchies and establishing the validity of each one, we will simply
consider one in one of the highest levels of human affairs, namely
education. In other words, we will accept the bridge between the
material and the abstract world. We will call it the Educational
Digestor. We will then observe how apt the correspondence is between
the crystal and the educational digestors. Von Bertalanffy has
already anticipated in general systems theory how important such
correspondences (isomorphisms, adjunctions, metaphors) will become.
Chomsky and Prigogine (1980) are also very sensitive to this problem.
We will first describe the educational digestor in terms of the
Didaskein. The Didaskein is a concept developed by the Pretoria
school of phenomenological educationalists (Van der Stoep and Louw).
We must remember that the Didaskein was developed in the apartheid era
when some laws actually made revolutionary (marxist) thinking (far
from equilbrium) a crime against the state. Thus the majority of white
people, even in education, gradually migrated to a kind of thinking
very close to equilibrium. The development of the Didaskein is a
typical example of this evolutionary (low entropy production)
thinking. Fourteen essential properties (categories) of the Didaskein
have been identified. Each of these essential properties may be
discussed as has been done by Van der
Stoep and Louw without any knowledge or reference to the crystal
digestor. What we now have to do to set up a correspondence, is to
observe whether it is also possible to discuss them sensibly by using
the crystal digestor as metaphor. Conversely, we must observe whether
it is possible to describe Ostwald's digestion process sensibly by
using terminology refering to human learning.
Such descriptions are not only possible, but are also metaphorically
sensible. (I will not do it here because it will involve many screens
of descriptions.) Once we make sure that this isomorphism
(correspondence, adjunction) does make sense, we have established a
powerful categorical identity (see the essentiality sureness.) We may
then speak of Digestors in general rather than typical digestors such
as the Crystal Digestor or the Educational Digestor. Furthermore, we
also have a new way of introducing and understanding dynamical
quantities such as enegry E, entropy S and free energy F, originally
refering to the material world of brain, to the abtsract world of
mind.
Dan, what you now have to do, is to play with the Digestor in your
mind, trying to perceive possible applications. Let me use the
"tyranny of the experts" as an example because you have made an
intuitive connection between it and the Digestor. Experts are like
crystals in the surroundings SU. They are experts because they know
much about their field, i.e a high mSU value. But the majority of them
know very little about other vastly different topics and the network
of relationships between such disciplines. Thus they are low in Msu
values. Their tyranny or intimidation is experienced by the system
(me, you or anybody else not an expert in their field) when the system
is low in mSY or Msy.
By becoming self an expert, the system increase its mSY value and
hence is empowered to a greater region of usable free energy (darker
coloured areas on the graphs). But the system can also increase its
Msy by experiencing a rich variety of emergences leading to higher
orders. Thus the system need not to be so large in mSY to maintain its
spontaneous self-organisation. We now see why quality in models of
system dynamics such as TQM (Total Quality Management) can have such a
remarkable improvement on the functioning of the organisation. What
TQM does, is to pull the Digestor graph downwards (moving from graph 1
with Msy<Msu to graph 3 with Msy>Msu) so that the system covers a
larger darker coloured area on the graph, i.e.more free energy
available for organisational purposes.
Dan, remember that the free energy F is that part of the total enegy E
available to the system by which it can change its future organisation
in terms of increased chaos or order. Whether the system uses this
free energy wisely (emergences far form equilbrium or digestions close
to equilbrium) is another issue. To go for an emergence when the
system need a digestion or for evolution when it needs a revolution,
is a foolish thing to do. Take once again South Africa as example. The
ruling party during the era of apartheid outlawed revolutionary (far
from equilibrium) thinking. Thus it outlawed its only hope of escaping
the ideology of apartheid. In other words, it was easy to grow in mSY,
but very difficult in Msy. If we prevent the germination of seed, the
forest will be destroyed eventually because no saplings are availble
to replace the old trees used for fire wood.
Doing the opposite as in the former communistic countries, namely
outlawing evolutionary thoughts against the ideology of the eternal
revolution, decreases the opportunities for digestion. A revolutionary
thought needs opportunities to digest into maturity before it can act
as a new source of free energy for a different organisational change.
If we keep on using saplings for fire wood rather than well aged
trees, the forest eventually will also become destroyed.
Best wishes
--At de Lange <amdelange@gold.up.ac.za> Snailmail: A M de Lange Gold Fields Computer Centre Faculty of Science - University of Pretoria Pretoria 0001 - Rep of South Africa
Learning-org -- Hosted by Rick Karash <rkarash@karash.com> Public Dialog on Learning Organizations -- <http://www.learning-org.com>